Beniamin BOGOSEL

Optimization of Supports in Additive Manufacturing

This work is in collaboration with Grégoire Allaire and Martin Bihr.

This is a companion webpage for the article: Support optimization in additive manufacturing for geometric and thermo-mechanical constraints.

Below you will find a recap of the results shown in the paper together with most of the data required to redo the computations. For the geometric methods a triangulation of the surface of $\partial \omega$ is given. For the results of the topology optimization algorithms the mesh, parameters, initialization and final results are shown.

Geometric methods

Below are the files used in the optimization of the orientation using geometric criteria. These are Matlab .mat files containing a structure with fields (among others):

• "points": containing the coordinates of the points
• "t": containing the triangulation as indices in the points array

• mesh of the torus
• mesh of a bolt
• mesh of a bunny
• mesh of the U-tube

Shape and Topology Optimization

The images below are created with the software xd3d by François Jouve.

U-shaped Tube

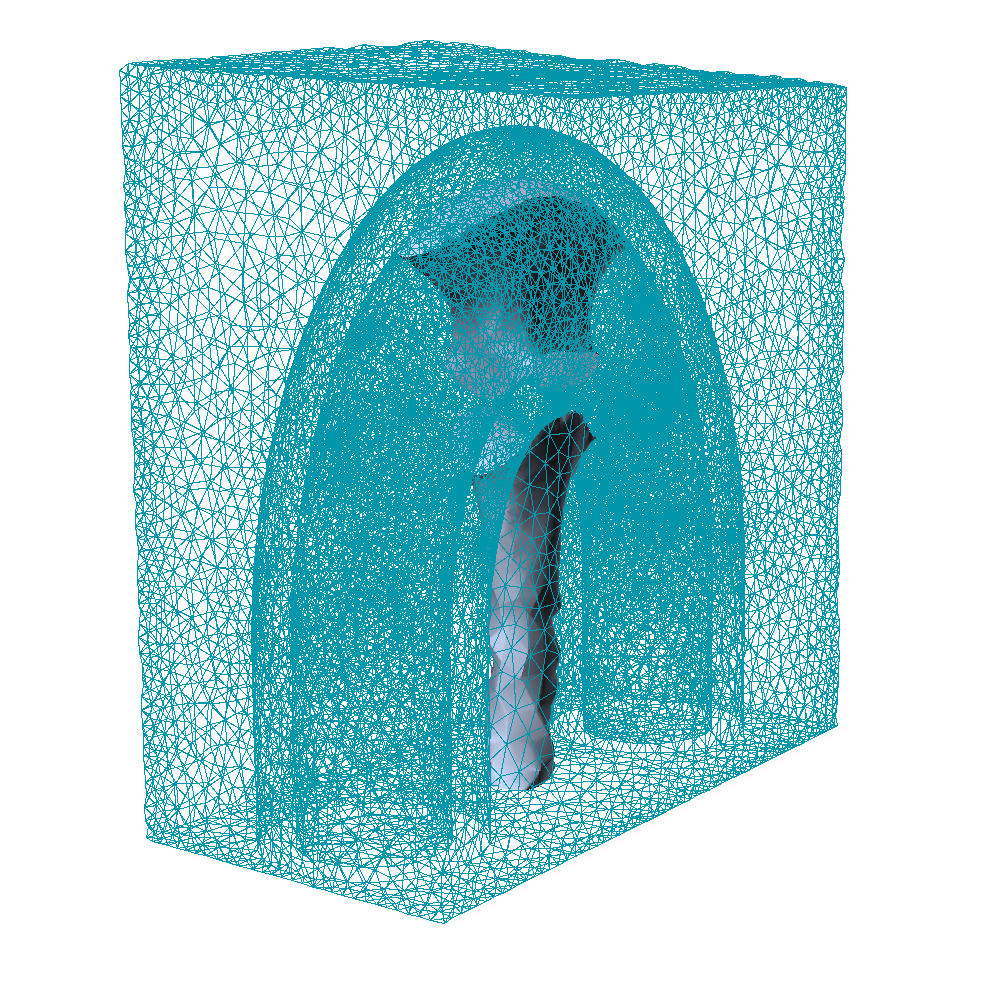
	The support may touch the shape $\omega$ mesh file initialization final result
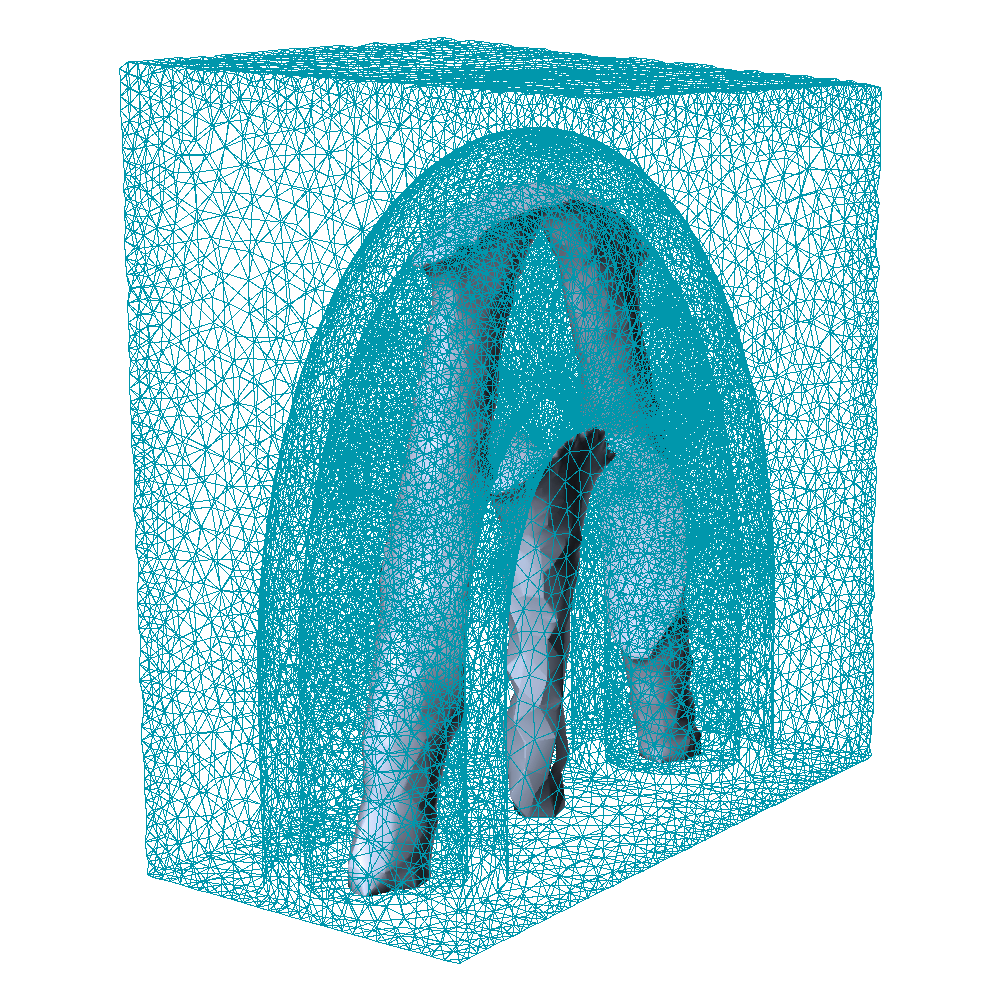
	Remove all Dirichlet conditions from $\partial\omega$: the support may still touch the shape mesh file initialization final result
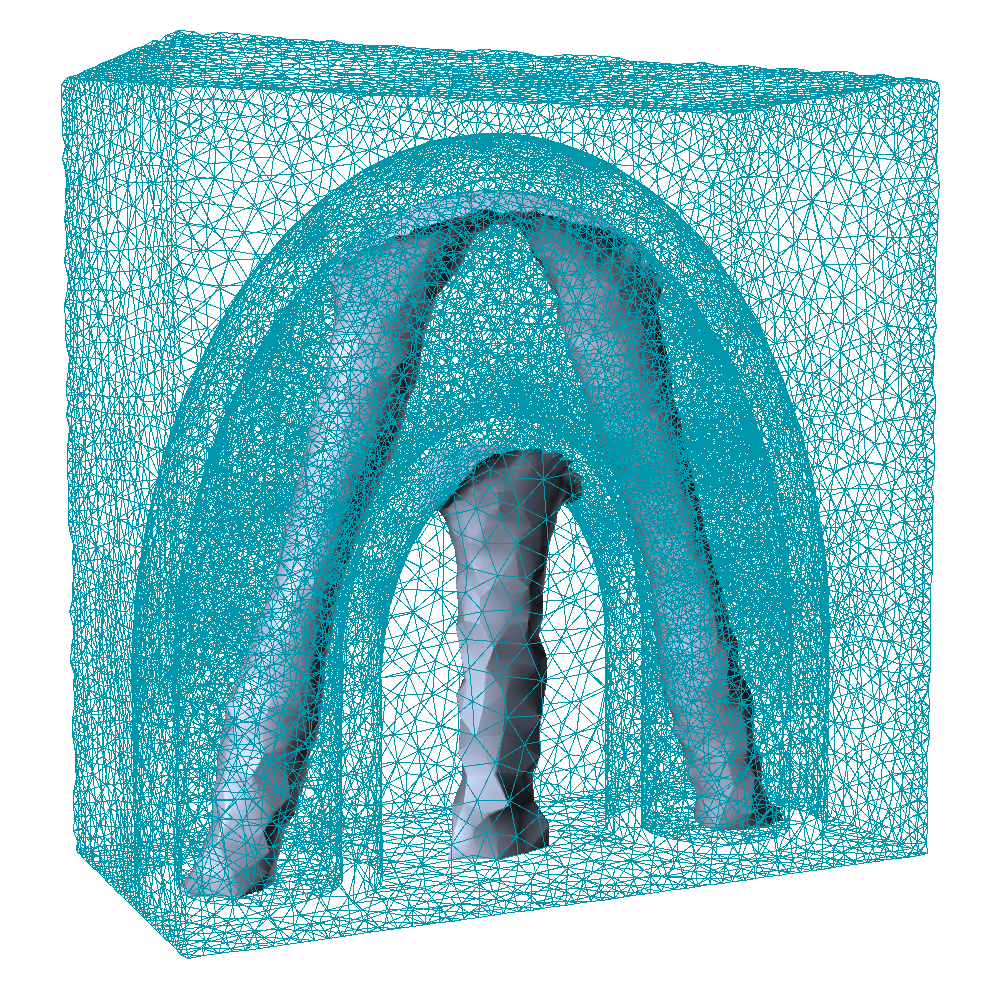
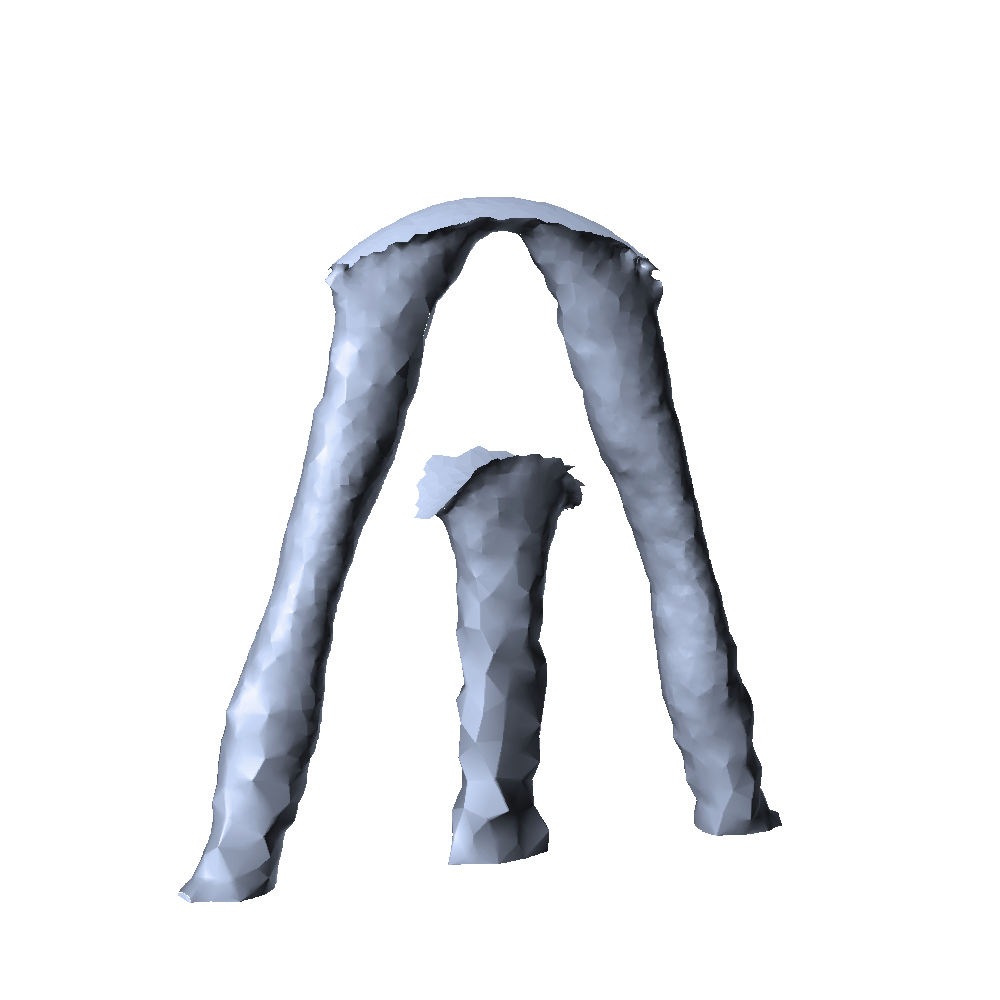
	Remove all Dirichlet conditions from $\partial\omega$ and add penalization term. The support does not touch the part! mesh file initialization final result

Three half U-tubes

The objective here is to obtain common supports for the same shape, repeated three times.

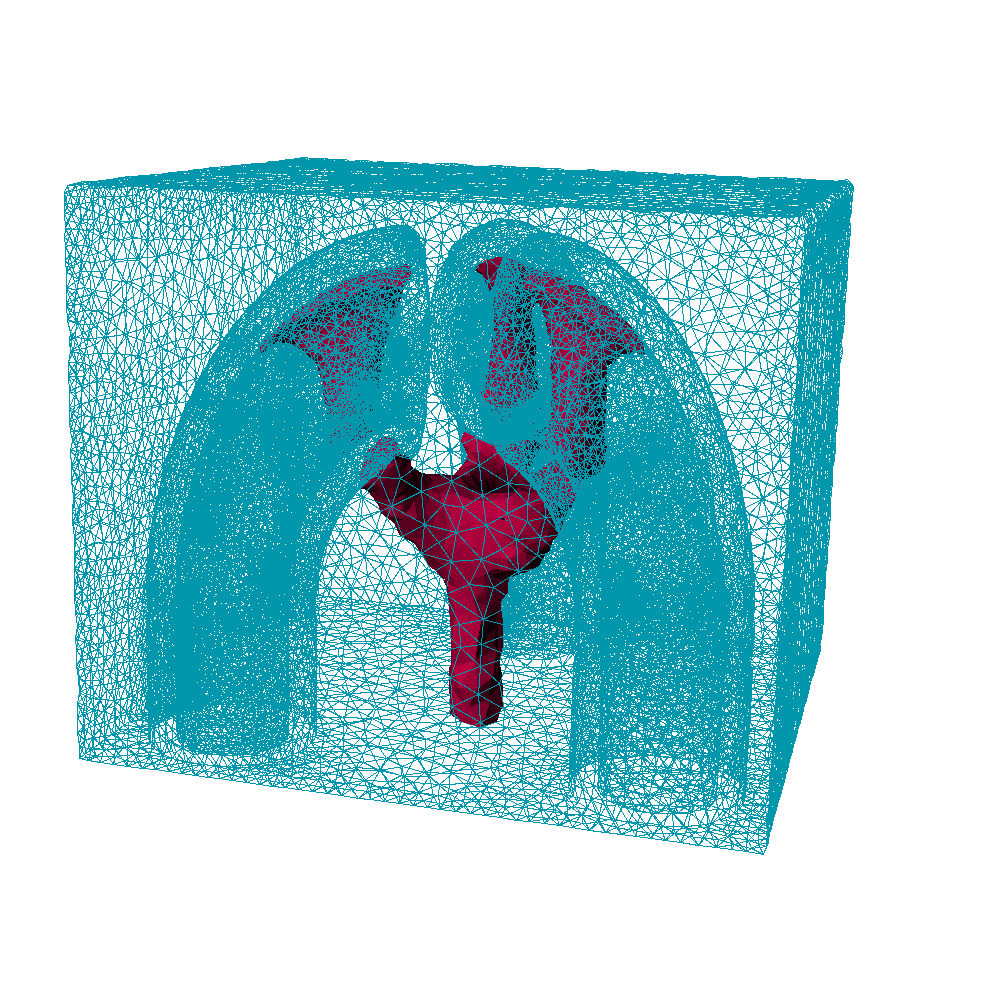
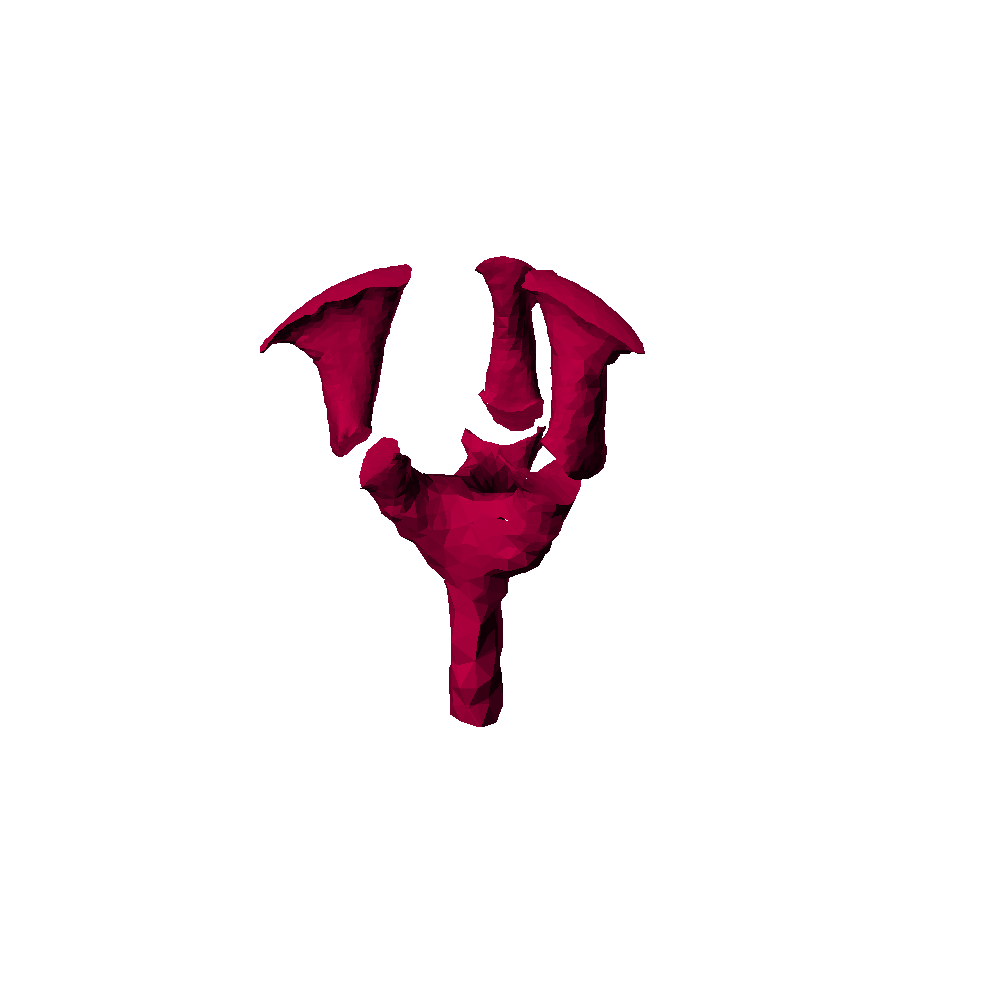
	The support may touch the shape mesh file initialization final result
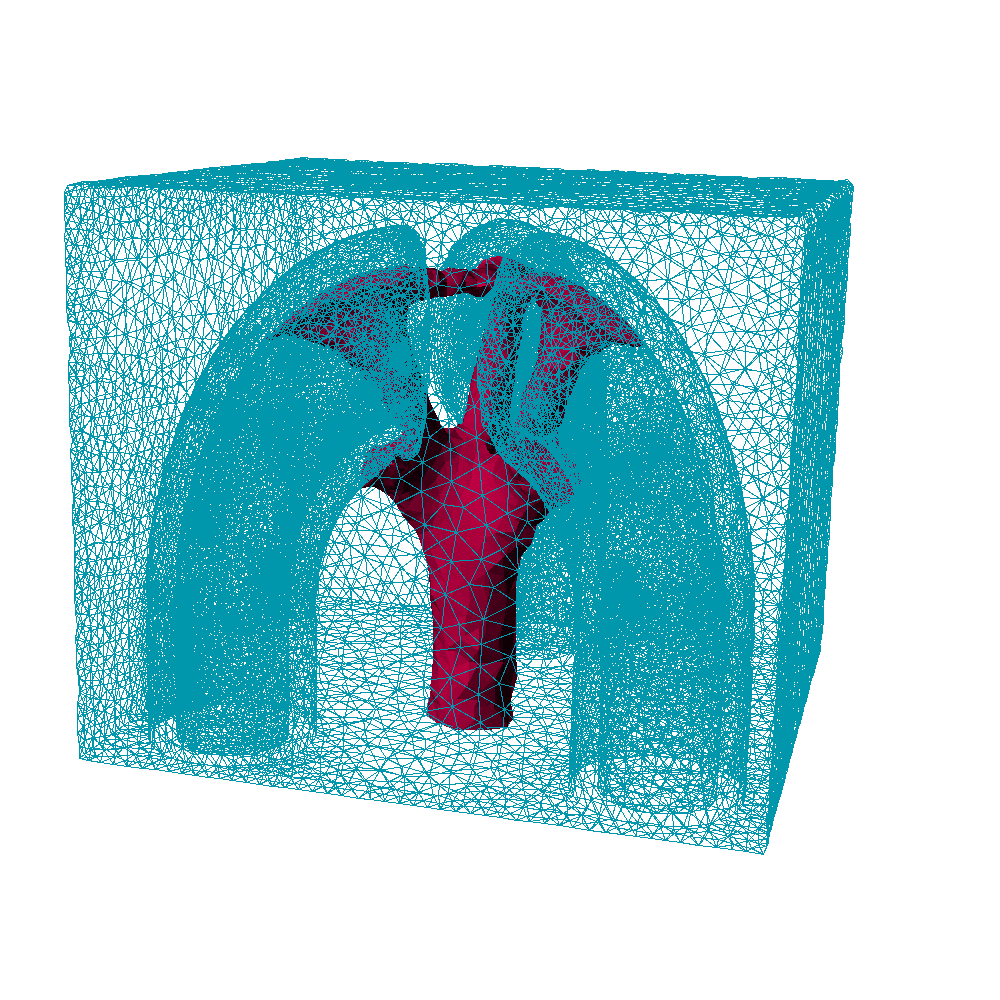
	Remove all Dirichlet conditions from $\partial\omega$: the support may still touch the shape mesh file initialization final result

---

Created: Sept 2019, Last modified: Sept 2019