

|
|
|
|

|

|
| Abstract: We establish the scaling limit of the geodesics to the root for the first passage percolation distance on random planar maps. We first describe the scaling limit of the number of faces along the geodesics. This result enables to compare the metric balls for the first passage percolation and the dual graph distance. It also enables to upperbound the diameter of large random maps. Then, we describe the scaling limit of the tree of first passage percolation geodesics to the root via a stochastic coalescing flow of pure jump diffusions. This stochastic flow also enables us to construct some random metric spaces which we conjecture to be the scaling limit of random planar maps with high degrees. The main tool in this work is a time-reversal of the uniform peeling exploration. |
|
| Abstract: We prove that for \(n=2\) the gaskets of critical rigid \(O(n)\) loop-decorated random planar maps are \(3/2\)-stable maps. The case \(n=2\) thus corresponds to the critical case in random planar maps. The proof relies on the Wiener-Hopf factorisation for random walks. Our techniques also provide a characterisation of weight sequences of critical \(O(2)\) loop-decorated maps. |
|
| Abstract: We consider large uniform random trees where we fix for each vertex its degree and height. We prove, under natural conditions of convergence for the profile, that those trees properly renormalized converge. To this end, we study the paths from random vertices to the root using coalescent processes. As an application, we obtain scaling limits of Bienaymé-Galton-Watson trees in varying environment. |
|
| Abstract: The purpose of this article is threefold. First, we show that when one explores a conformal loop ensemble of parameter \( \kappa=4\) (\( \mathrm{CLE}_4 \)) on an independent \(2\)-Liouville quantum gravity (\(2\)-LQG) disk, the surfaces which are cut out are independent quantum disks. To achieve this, we rely on approximations of the explorations of a \(\mathrm{CLE}_4\): we first approximate the \( \mathrm{SLE}^{\langle\mu \rangle }_4(-2) \) explorations for \(\mu \in \mathbb{R}\) using explorations of the \(\mathrm{CLE}_\kappa\) as \(\kappa \uparrow 4 \) and then we approximate the uniform exploration by letting \(\mu \to \infty\). Second, we describe the relation between the so-called natural quantum distance and the conformally invariant distance to the boundary introduced by Werner and Wu. Third, we establish the scaling limit of the distances from the boundary to the large faces of \(3/2\)-stable maps and relate the limit to the \(\mathrm{CLE}_4\)-decorated \(2\)-LQG. |
|
| Abstract: We investigate scaling limits of trees built by uniform attachment with freezing, which is a variant of the classical model of random recursive trees introduced in a companion paper. Here vertices are allowed to freeze, and arriving vertices cannot be attached to already frozen ones. We identify a phase transition when the number of non-frozen vertices roughly evolves as the total number of vertices to a given power. In particular, we observe a critical regime where the scaling limit is a random compact real tree, closely related to a time non-homogenous Kingman coalescent process identified by Aldous. Interestingly, in this critical regime, a condensation phenomenon can occur. |
|
| Abstract: In the classical model of random recursive trees, trees are recursively built by attaching new vertices to old ones. What happens if vertices are allowed to freeze, in the sense that new vertices cannot be attached to already frozen ones? We are interested in the impact of freezing on the height of such trees. | 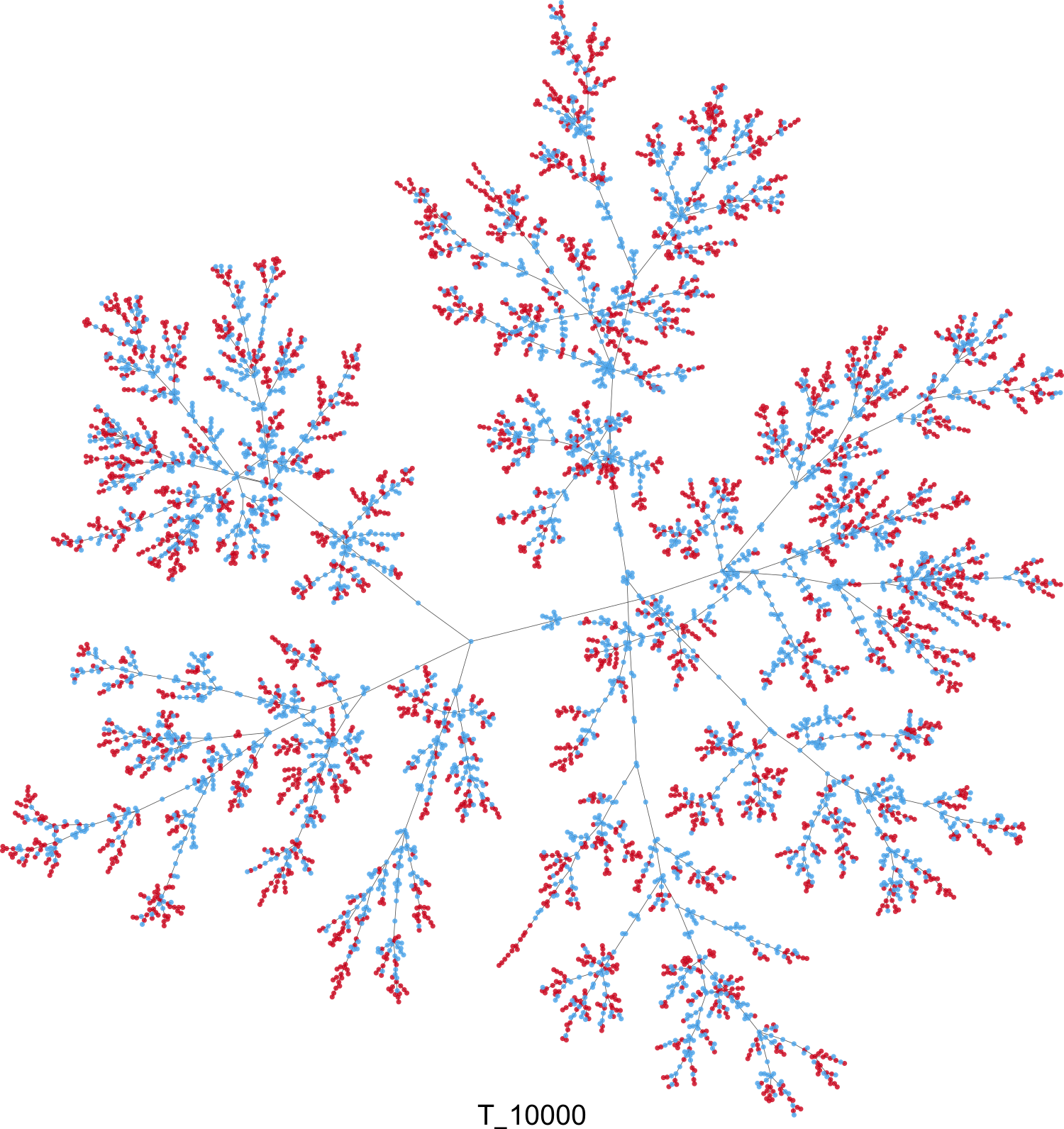 |
| Abstract: We discuss asymptotics of large Boltzmann random planar maps such that every vertex of degree \(k\) has weight of order \(k^{−2}\). Infinite maps of that kind were studied by Budd, Curien and Marzouk. These maps can be seen as the dual of the discrete \(\alpha\)-stable maps studied by Le Gall and Miermont for \(\alpha=3/2\) or as the gaskets of critical \(O(2)\)-decorated random planar maps. We compute the asymptotics of the graph distance and of the first passage percolation distance between two uniform vertices, which are respectively equivalent in probability to \((\log \ell)^2/\pi^2\) and \(2(\log \ell)/(\pi^2p_{\bf q})\) when the perimeter of the map \(\ell\) goes to \(\infty\), where \(p_{\bf q}\) is a constant which depends on the model. We also show that the diameter is of the same order as those distances for both metrics and obtain in particular that these maps do not satisfy scaling limits in the sense of Gromov-Prokhorov or Gromov-Hausdorff for lack of tightness. To study the peeling exploration of these maps, we prove local limit and scaling limit theorems for a class of random walks with heavy tails conditioned to remain positive until they die at \(-\ell\) towards processes that we call stable Lévy processes conditioned to stay positive until they jump and die at \(−1\). | 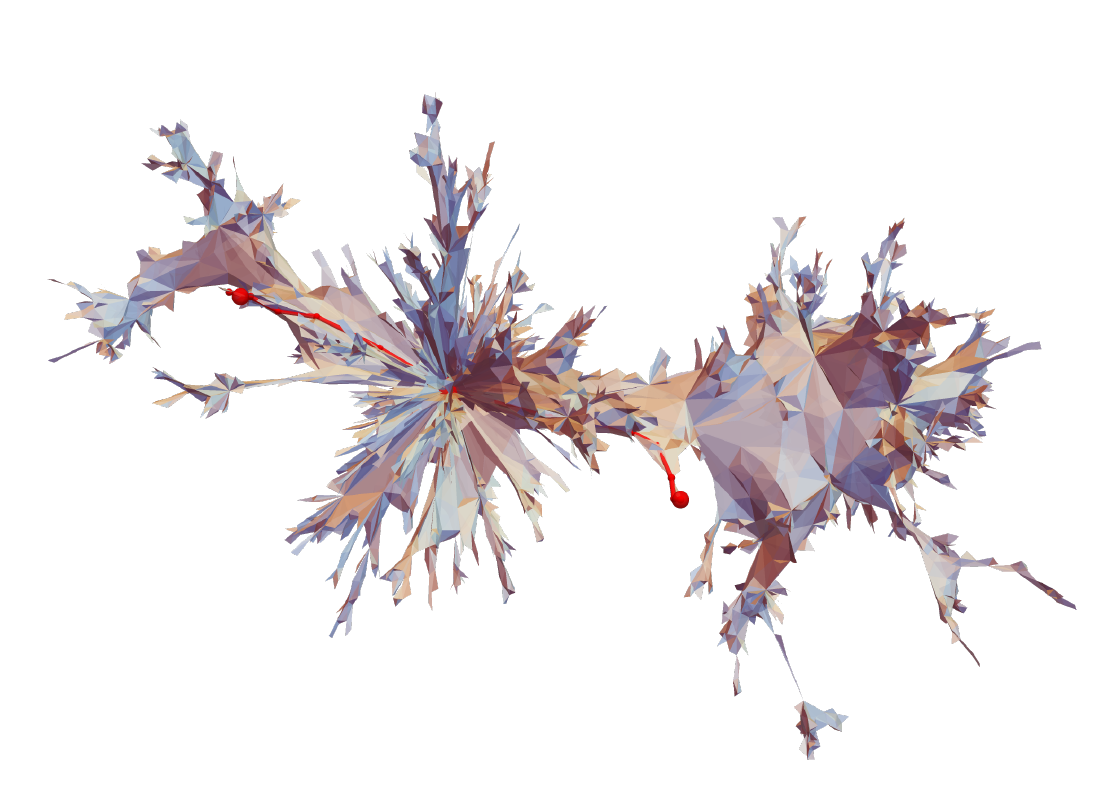 |