 |
    |
On the Behaviour of the (1,
)-
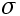SA-ES for a Constrained Linear Problem
Dirk V. Arnold
Faculty of Computer Science, Dalhousie University Halifax, Nova Scotia, Canada B3H 4R2dirk@cs.dal.ca
Abstract. This paper analyses the behaviour of the (1,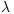
)-
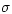SA-ES with deterministic two-point rule when applied to a linear problem with a single linear constraint. Equations that describe the single-step behaviour of the strategy are derived and then used to predict the strategy’s multi-step behaviour. The findings suggest that mutative self-adaptation will result in convergence of the (1,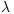)-ES to non-stationary points if the angle between the gradient vector of the objective function and the normal vector of the constraint plane is small. Comparisons with the behaviour of evolution strategies that employ other step size adaptation mechanisms are drawn.
LNCS 7491, p. 82 ff.
lncs@springer.com
© Springer-Verlag Berlin Heidelberg 2012